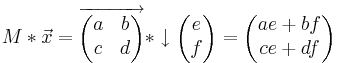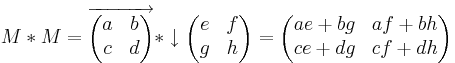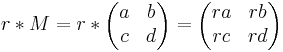Affine Abbildungen und deren Eigenschaften.: Unterschied zwischen den Versionen
Aus KAS-Wiki
Georg1 (Diskussion | Beiträge) |
Georg1 (Diskussion | Beiträge) |
||
| Zeile 8: | Zeile 8: | ||
Affine Abbildungen werden im zweidimensionelem Raum durch 2X2 Matrizen ausgedrückt. Sie haben die Form <math>\begin{pmatrix} | Affine Abbildungen werden im zweidimensionelem Raum durch 2X2 Matrizen ausgedrückt. Sie haben die Form <math>\begin{pmatrix} | ||
| − | a & c \\ | + | a & b \\ |
| − | b & d | + | c & d |
| − | \end{pmatrix}</math> | + | \end{pmatrix}</math>. Für die affinen Abbildungen wichtige Rechenoperationen sind vor allem die Multiplikationen. Dabei gelten folgende Regeln: |
| + | |||
| + | <math>M*\vec x=\overrightarrow\begin{pmatrix} a & b \\ c & d \end{pmatrix}*\downarrow\begin{pmatrix} e \\ f \end{pmatrix}=\begin{pmatrix} ae+bf \\ ce+df \end{pmatrix}</math> | ||
| + | |||
| + | <math>M*M=\overrightarrow\begin{pmatrix} a & b \\ c & d \end{pmatrix}*\downarrow\begin{pmatrix} e & f \\ g & h \end{pmatrix}=\begin{pmatrix} ae+bg & af+bh \\ ce+dg & cf+dh \end{pmatrix}</math> | ||
| + | |||
| + | <math>r*M=r*\begin{pmatrix} a & b \\ c & d \end{pmatrix}=\begin{pmatrix} ra & rb \\ rc & rd \end{pmatrix}</math> | ||
Version vom 7. Dezember 2009, 11:18 Uhr
Affine Abbildungen
Definition
Affine Abbildungen sind geometrische Projektionen. Mit Hilfe von Abbildungsmatrizen wird ein geometrischer Prozess wie z.B. Spiegelungen, Drehungen um beliebige Zentren, Streckungen oder Stauchungen mathematisch erfasst und beschrieben. Um mit ihnen umgehen zu können bedarf es der Rechenregeln für Matrizen.
Matrizenrechnung
Affine Abbildungen werden im zweidimensionelem Raum durch 2X2 Matrizen ausgedrückt. Sie haben die Form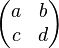 
. Für die affinen Abbildungen wichtige Rechenoperationen sind vor allem die Multiplikationen. Dabei gelten folgende Regeln:
