Ableitungsregeln.: Unterschied zwischen den Versionen
Aus KAS-Wiki
Vieth1 (Diskussion | Beiträge) (→Beispiel) |
K (→Differenzregel) |
||
| (16 dazwischenliegende Versionen von 3 Benutzern werden nicht angezeigt) | |||
| Zeile 11: | Zeile 11: | ||
<math>f\!(x)=x^2</math> | <math>f\!(x)=x^2</math> | ||
| − | => <math>f\!\,'(x)=2x^{2-1}=</math><math>\!\,2x</math> | + | => <math>f\!\,'(x)=2x^{2-1}=</math><math>\!\,\boldsymbol{\underline{\underline{2x}}}</math> |
=== Summenregel === | === Summenregel === | ||
| Zeile 24: | Zeile 24: | ||
<math>f\!(x)=x^3+x^2</math> | <math>f\!(x)=x^3+x^2</math> | ||
| − | => <math>f\!\,'(x)= (x^3)' + (x^2)' = 3x^2 + 2x </math> | + | => <math>f\!\,'(x)= (x^3)' + (x^2)' = \boldsymbol{\underline{\underline{3x^2 + 2x}}} </math> |
| Zeile 40: | Zeile 40: | ||
<math>f\!(x)=6x^5</math> | <math>f\!(x)=6x^5</math> | ||
| − | => <math>f\!\,'(x)=6*5x^{(5-1)} = 30x^4 </math> | + | => <math>f\!\,'(x)=6*5x^{(5-1)} = \boldsymbol{\underline{\underline{30x^4}}} </math> |
| Zeile 49: | Zeile 49: | ||
<math>f\!(x)=g(x)-h(x)</math> | <math>f\!(x)=g(x)-h(x)</math> | ||
| − | => <math>f\!\,'(x)=g'(x)-h'( | + | => <math>f\!\,'(x)=g'(x)-h'(x)</math> |
| Zeile 56: | Zeile 56: | ||
<math>f\!(x)=x^9-x^7</math> | <math>f\!(x)=x^9-x^7</math> | ||
| − | => <math>f\!\,'(x)= (x^9)' - (x^7)' = 9x^8 | + | => <math>f\!\,'(x)= (x^9)' - (x^7)' = \boldsymbol{\underline{\underline{9x^8 - 7x^6}}} </math> |
| − | + | ||
| − | + | ||
=== Produktregel === | === Produktregel === | ||
| Zeile 93: | Zeile 91: | ||
| − | |||
| − | + | ==== Beispiel 2 ==== | |
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| − | + | ||
| Zeile 135: | Zeile 113: | ||
<math>f\!\,'(x)=\boldsymbol{\underline{\underline{x^{n-1} \cdot e^{x} \cdot (n+x)}}}</math> | <math>f\!\,'(x)=\boldsymbol{\underline{\underline{x^{n-1} \cdot e^{x} \cdot (n+x)}}}</math> | ||
| − | |||
| − | |||
=== Quotientenregel === | === Quotientenregel === | ||
| Zeile 173: | Zeile 149: | ||
=== Kettenregel === | === Kettenregel === | ||
| − | <math>f\!(x)=g(h(x))</math> | + | <math>f\!(x)=g(h(x))</math> bzw. <math>f\!(x)=g(u)</math> mit <math>u=h\!(x)</math> |
| − | => <math>f\!\,'(x)=h'( | + | => <math>f\!\,'(x)=h'(u) \cdot g'(h(x))</math> |
| + | Die Ableitung von einer verketteten Funktion wird grob gesagt gebildet, indem man erst die äußere Ableitung und dann die innere bildet. | ||
==== Beispiel ==== | ==== Beispiel ==== | ||
| + | <math>f\!(x) = (x^2 + 2x)^2</math> <br /> | ||
| + | <math>f\! '(x) = 2(x^2 + 2x)*(2x + 2) </math> | ||
| + | |||
| + | ==== Hilfestellung ==== | ||
| + | |||
| + | <math>f\!(x) = u(v(x))</math><br /> | ||
| + | <math>f\! '(x) = u'(v(x)) * v'(x)</math> | ||
| + | |||
| + | '''Beispiel von oben:''' | ||
| + | <math>f\! (x) = (x^2 + 2x)^2</math> | ||
| + | |||
| + | <math>u\! = ()^2</math><math>u\! ' = 2()</math> <br /> | ||
| + | <math>v\! = x^2+2x </math><math>v\! ' = 2x+2</math> | ||
| + | |||
| + | |||
| + | <math>f\! '(x) =2(x^2 + 2x)*(2x + 2) </math><br /> | ||
| + | Also: <math>f\! '(x) = u' (v(x)) * v'(x)</math> | ||
=== Umkehrregel === | === Umkehrregel === | ||
| − | <math> | + | <math>x=g(y)\ Umkehrfunktion\ von\ y=f(x)</math> |
| − | <math> | + | <math>=>\ g'(y)= \frac{1}{f'(x)}</math> |
| + | === Ableitungen (Ableitungsfunktionen) spezieller Funktionen === | ||
| − | |||
| + | {| class="wikitable center" | ||
| + | |- style="background: #DDFFDD;" | ||
| + | ! <math>\!f(x)</math> | ||
| + | ! <math>\!f'(x)</math> | ||
| + | ! <math>\!f''(x)</math> | ||
| + | |- | ||
| + | | <math>\!a= konstante Zahl</math> | ||
| + | | <math>\!0</math> | ||
| + | | <math>\!0</math> | ||
| + | |- | ||
| + | | <math>\!x^n</math> | ||
| + | | <math>\!nx^{(n-1)}</math> | ||
| + | | <math>\!n(n-1)x^{(n-2)}</math> | ||
| + | |- | ||
| + | | <math>\sqrt{x}</math> | ||
| + | | <math>\frac{1}{2\sqrt{x}}</math> | ||
| + | | <math>-\frac{1}{4x\sqrt{x}}</math> | ||
| + | |- | ||
| + | | <math>\!e^x</math> | ||
| + | | <math>\!e^x</math> | ||
| + | | <math>\!e^x</math> | ||
| + | |- | ||
| + | | <math>\!a^x</math> | ||
| + | | <math>\!a^x*ln\ a</math> | ||
| + | | <math>\!a^x*(ln\ a)^2</math> | ||
| + | |- | ||
| + | | <math>\! log_a x</math> | ||
| + | | <math>\! \frac{1}{x*ln\ a}</math> | ||
| + | | <math>\! \frac{-1}{x^2*ln\ a}</math> | ||
| + | |- | ||
| + | | <math>\! ln\ x</math> | ||
| + | | <math>\! \frac{1}{x}</math> | ||
| + | | <math>\! -\frac{1}{x^2}</math> | ||
| + | |- | ||
| + | |} | ||
| − | + | [[Kategorie:Funktionen]] | |
Aktuelle Version vom 9. Dezember 2013, 09:33 Uhr
Inhaltsverzeichnis |
Ableitungsregeln
Potenzregel
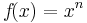
=> 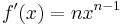
Beispiel

=> 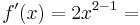
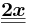
Summenregel

=> 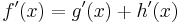
Beispiel

=> 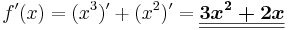
Faktorregel
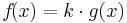
=> 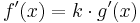
Beispiel
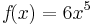
=> 
Differenzregel

=> 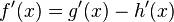
Beispiel
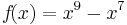
=> 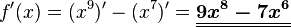
Produktregel
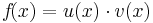
=> 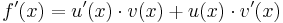
Beispiel 1
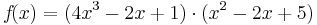
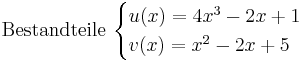
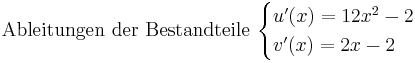
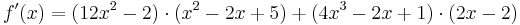
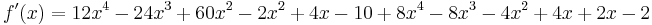

Beispiel 2
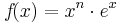

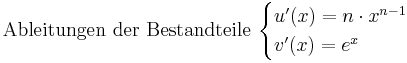
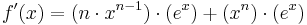
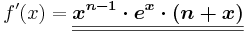
Quotientenregel

=> 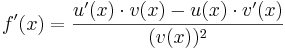
Beispiel
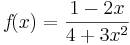
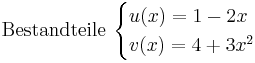
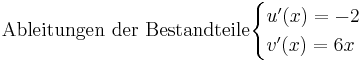
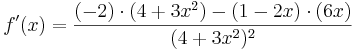
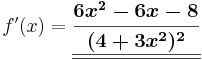
Kettenregel
 bzw.
bzw.  mit
mit 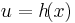
=> 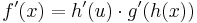
Die Ableitung von einer verketteten Funktion wird grob gesagt gebildet, indem man erst die äußere Ableitung und dann die innere bildet.
Beispiel
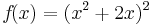
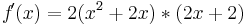
Hilfestellung

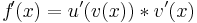
Beispiel von oben:
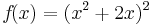
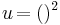
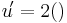
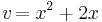
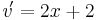
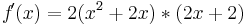
Also: 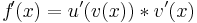
Umkehrregel


Ableitungen (Ableitungsfunktionen) spezieller Funktionen
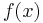
|

|
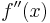
|
|---|---|---|

|
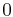
|
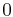
|
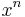
|
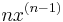
|
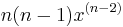
|
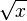
|
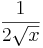
|
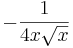
|
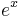
|
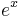
|
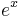
|
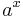
|
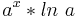
|
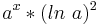
|
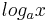
|
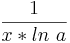
|
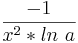
|
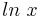
|
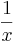
|
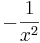
|

