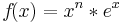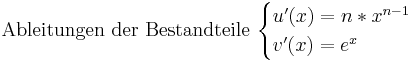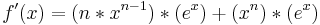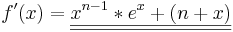Ableitungsregeln: Unterschied zwischen den Versionen

Aus KAS-Wiki
Wechseln zu: Navigation, Suche
Zeile 96: Zeile 96:
  
 
<math>f\!\,'(x)=\underline{\underline{20x^4-32x^3+54x^2+10x-12}}</math>
 
<math>f\!\,'(x)=\underline{\underline{20x^4-32x^3+54x^2+10x-12}}</math>
 +
 +
 +
 +
==== Beispiel 2 ====
 +
 +
 +
<math>f\!(x)=\sin(x)*\cos(x)</math>
 +
 +
<math>\mathrm{Bestandteile}\ \begin{cases}
 +
u(x)=\sin(x)\\
 +
v(x)=\cos(x)
 +
\end{cases}</math>
 +
 +
 +
<math>\mathrm{Ableitungen\ der\ Bestandteile}\ \begin{cases}
 +
u'(x)=\cos(x)\\
 +
v'(x)=-\sin(x)
 +
\end{cases}</math>
 +
 +
 +
<math>f\!\,'(x)=\cos(x)*\cos(x)+\sin(x)*(-\sin(x))</math>
 +
 +
<math>f\!\,'(x)=\underline{\underline{\cos^2(x)-\sin^2(x)}}</math>
 +
 +
 +
==== Beispiel 3 ====
 +
 +
 +
<math>f\!(x)=x^n*e^{x}</math>
 +
 +
<math>\mathrm{Bestandteile}\ \begin{cases}
 +
u(x)=x^n\\
 +
v(x)=e^{x}
 +
\end{cases}</math>
 +
 +
 +
<math>\mathrm{Ableitungen\ der\ Bestandteile}\ \begin{cases}
 +
u'(x)=n*x^{n-1}\\
 +
v'(x)=e^{x}
 +
\end{cases}</math>
 +
 +
 +
<math>f\!\,'(x)=(n*x^{n-1})*(e^{x})+(x^n)*(e^{x})</math>
 +
 +
<math>f\!\,'(x)=\underline{\underline{x^{n-1}*e^{x}+(n+x)}}</math>

Version vom 1. Dezember 2009, 13:24 Uhr

Inhaltsverzeichnis

Ableitungsregeln

Potenzregel

f\!(x)=x^n

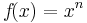
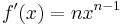
=> f\!\,'(x)=nx^{n-1}


Summenregel

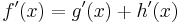
f\!(x)=g(x)+h(x)

=> f\!\,'(x)=g'(x)+h'(x)


Faktorregel

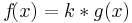
f\!(x)=k*g(x)

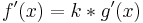
=> f\!\,'(x)=k*g'(x)


Differenzregel

f\!(x)=g(x)-h(x)

=> f\!\,'(x)=g'(x)-h'(h)


Produktregel

f\!(x)=u(x)*v(x)

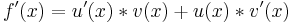
=> f\!\,'(x)=u'(x)*v(x)+u(x)*v'(x)


Quotientenregel

f\!(x)=\frac{u(x)}{v(x)}


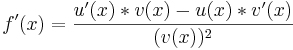
=> f\!\,'(x)=\frac{u'(x)*v(x)-u(x)*v'(x)}{(v(x))^2}


Kettenregel

f\!(x)=g(h(x))

=> f\!\,'(x)=h'(x)*g'(h(x))


Umkehrregel

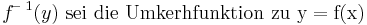
f\!^-\,^1(y)\ \mathrm{sei\ die\ Umkerhfunktion\ zu\ y=f(x)}

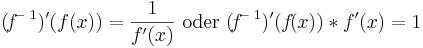
(\!f\!^-\,^1)'(f(x))=\frac{1}{f'(x)}\ \mathrm{oder}\ (\!f\!^-\,^1)'(f\!(x))*f\!\,'(x)=1


Beispiele

Summenregel

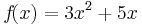
f\!(x)=3x^2+5x

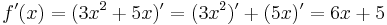
=> f\!\,'(x)=(3x^2+5x)'=(3x^2)'+(5x)'=6x+5


Produktregel

Beispiel 1

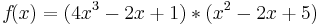
f\!(x)=(4x^3-2x+1)*(x^2-2x+5)


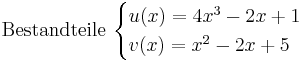
\mathrm{Bestandteile}\ \begin{cases} u(x)=4x^3-2x+1\\ v(x)=x^2-2x+5 \end{cases}


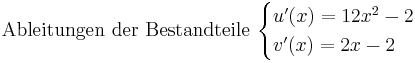
\mathrm{Ableitungen\ der\ Bestandteile}\ \begin{cases} u'(x)=12x^2-2\\ v'(x)=2x-2 \end{cases}


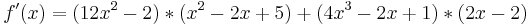
f\!\,'(x)=(12x^2-2)*(x^2-2x+5)+(4x^3-2x+1)*(2x-2)

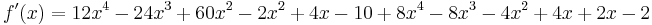
f\!\,'(x)=12x^4-24x^3+60x^2-2x^2+4x-10+8x^4-8x^3-4x^2+4x+2x-2

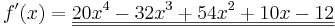
f\!\,'(x)=\underline{\underline{20x^4-32x^3+54x^2+10x-12}}


Beispiel 2

f\!(x)=\sin(x)*\cos(x)

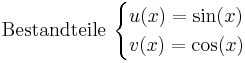
\mathrm{Bestandteile}\ \begin{cases} u(x)=\sin(x)\\ v(x)=\cos(x) \end{cases}


\mathrm{Ableitungen\ der\ Bestandteile}\ \begin{cases} u'(x)=\cos(x)\\ v'(x)=-\sin(x) \end{cases}


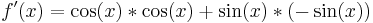
f\!\,'(x)=\cos(x)*\cos(x)+\sin(x)*(-\sin(x))

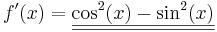
f\!\,'(x)=\underline{\underline{\cos^2(x)-\sin^2(x)}}


Beispiel 3

f\!(x)=x^n*e^{x}

\mathrm{Bestandteile}\ \begin{cases} u(x)=x^n\\ v(x)=e^{x} \end{cases}


\mathrm{Ableitungen\ der\ Bestandteile}\ \begin{cases} u'(x)=n*x^{n-1}\\ v'(x)=e^{x} \end{cases}


f\!\,'(x)=(n*x^{n-1})*(e^{x})+(x^n)*(e^{x})

f\!\,'(x)=\underline{\underline{x^{n-1}*e^{x}+(n+x)}}

Von „http://kas.zum.de/index.php?title=Ableitungsregeln&oldid=288“