Affine Abbildungen: Unterschied zwischen den Versionen
Aus KAS-Wiki
(→Definition:) |
|||
| Zeile 25: | Zeile 25: | ||
{| class="wikitable" | {| class="wikitable" | ||
|- | |- | ||
| − | | Abbildung || Flächeninhalt || Fixpunkte, Fixgeraden | + | | Abbildung || Flächeninhalt || Fixpunkte, Fixgeraden || Matrixdarstellung |
|- | |- | ||
| − | | Spiegelung || A bleibt gleich || (0/0) Spiegelgerade (Fixgerade) | + | | Spiegelung || A bleibt gleich || (0/0) Spiegelgerade (Fixgerade) || <math>M=\begin{pmatrix} cos2\varphi & sin2\varphi \\ sin2\varphi & -cos2\varphi \end{pmatrix}</math> |
|- | |- | ||
| − | | zentrische Streckung || <math>A'= 3^2A</math> || (0/0) x-und y-Achse jede Gerade <math>y=a*x</math> | + | | zentrische Streckung || <math>\!A'= 3^2A</math> || (0/0) x-und y-Achse jede Gerade <math>y=a*x</math> || <math>M*\begin{pmatrix} k & 0 \\ 0 & k \end{pmatrix}</math> |
|- | |- | ||
| − | | Drehung || A bleibt gleich || (0/0) | + | | Drehung || A bleibt gleich || (0/0) || <math>M=\begin{pmatrix} cos\varphi & sin\varphi \\ -sin\varphi & cos\varphi \end{pmatrix}</math> |
|- | |- | ||
| − | | Skalierung || <math>A'=2*4A</math> || (0/0) x- und y-Achse | + | | Skalierung || <math>\!A'=2*4A</math> || (0/0) x- und y-Achse |
|- | |- | ||
| − | | Scherung || <math>A'=A</math> || (0/0) x- oder y-Achse | + | | Scherung || <math>\!A'=A</math> || (0/0) x- oder y-Achse |
|} | |} | ||
Version vom 7. Dezember 2009, 08:54 Uhr
Definition:
Eine geradentreue und umkehrbare geometrische Abbildung der Ebene auf sich selbst nennt man eine affine Abbildung oder Affinität.
Die affine Abbildung bildet ein neues Koordinatensystem.
Die einzelnen Spalten der Matrix dürfen nicht linear abhängig sein.
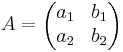
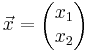


LGS:
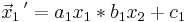
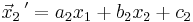
| Abbildung | Flächeninhalt | Fixpunkte, Fixgeraden | Matrixdarstellung |
| Spiegelung | A bleibt gleich | (0/0) Spiegelgerade (Fixgerade) | 
|
| zentrische Streckung | 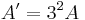 |
(0/0) x-und y-Achse jede Gerade 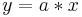 |
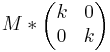
|
| Drehung | A bleibt gleich | (0/0) | 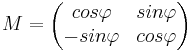
|
| Skalierung | 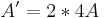 |
(0/0) x- und y-Achse | |
| Scherung | 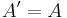 |
(0/0) x- oder y-Achse |

