Ganzrationale Funktionen.: Unterschied zwischen den Versionen
Aus KAS-Wiki
Franca (Diskussion | Beiträge) (→{ \color{Black} p/q-Formel:}) |
Franca (Diskussion | Beiträge) (→{ \color{Black} p/q-Formel:}) |
||
| Zeile 21: | Zeile 21: | ||
==== Nullstellen der Funktion:<br /><br /> f(x)=0 ==== | ==== Nullstellen der Funktion:<br /><br /> f(x)=0 ==== | ||
| − | == <math>{ \color{Black} p/q-Formel:}</math> == | + | == 1.)<math>{ \color{Black} p/q-Formel:}</math> == |
| Zeile 27: | Zeile 27: | ||
f(x) = <math>x^2</math> + px + q = 0 | f(x) = <math>x^2</math> + px + q = 0 | ||
| − | + | <math>x_{1,2}</math> = <math>\frac{-p}{2}</math> <math> \pm \sqrt{ (\frac{-p}{2})^2-q }</math> | |
* f(x)= ax<sup>2</sup> + bx + c | * f(x)= ax<sup>2</sup> + bx + c | ||
Version vom 14. Dezember 2009, 10:51 Uhr
Inhaltsverzeichnis |
Ganzrationale Funktionen
f(x) = anxn + an-1xn-1 + ... + a1x + a0
n = Grad des Polynoms
Definitionsbereich:
-
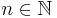
- an
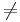0
Bestimmung einer ganzrationalen Funktion in Sachzusammenhängen:
- lineares Gleichungssystem aufstellen und lösen
- Koordinatensystem auswählen
- Bedingung: n + 1 Bedingungen sind nötig
Nullstellen der Funktion:
f(x)=0
1.)
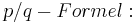
f(x) = 
+ px + q = 0
= 
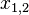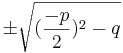
- f(x)= ax2 + bx + c
