Skalarprodukt, Vektorprodukt.: Unterschied zwischen den Versionen
Sama12 (Diskussion | Beiträge) |
Sama12 (Diskussion | Beiträge) |
||
| Zeile 58: | Zeile 58: | ||
und -11 ist<math>\not=</math> 0, deswegen sind<math> \vec a</math> und<math> \vec b</math> '''nicht orthogonal''' | und -11 ist<math>\not=</math> 0, deswegen sind<math> \vec a</math> und<math> \vec b</math> '''nicht orthogonal''' | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | '''Vektorprodukt''' oder auch '''Kreuzprodukt''' | ||
| + | |||
| + | Mit dem Vektorprodukt rechnet man den Vektor aus, der zu <math> \vec a</math> und<math> \vec b</math> '''orthogonal ist''' | ||
Version vom 14. Dezember 2009, 11:47 Uhr
Mit dem Skalarprodukt rechnet man den Winkel zwischen zwei Vektoren.
Die allgemeine Formel lautet : 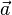 *
*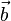 =
=  *
*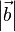 * cos
* cos 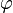
Um den Winkel zwischen zwei Vektoren auszurechnen formt man die Formel um in
cos=
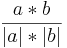
Beispiel 1:
Berechne den Winkel zwischen den Vektoren 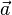 =
=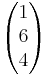 und
und 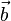 =
= 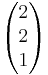
Also cos 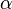 =
=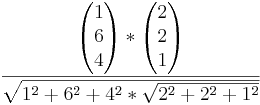 =
=  =
=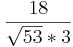 =
= daraus folgt
daraus folgt 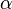
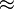 34,5°
34,5°
Orthogonalität
Wenn 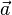 und
und 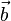 mit a
mit a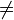 0 und b
0 und b 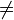 0 sind, dann gilt:
0 sind, dann gilt: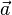 *
*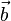 = 0
Gilt umgekehrt
= 0
Gilt umgekehrt 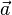 *
*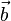 =0, dann sind
=0, dann sind 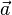 und
und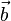 orthogonal.
orthogonal.
Beispiel 2
Prüfe,ob die Vektoren a=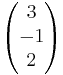 und b=
und b=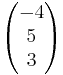 orthogonal (rechtwinklig) sind
orthogonal (rechtwinklig) sind
Bedingung ist 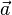 *
*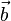 muss gleich 0 sein
muss gleich 0 sein
Also :
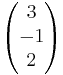 *
* = 3* (-4) + (-1) * 5 + 2 * 3= -11
= 3* (-4) + (-1) * 5 + 2 * 3= -11
und -11 ist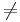 0, deswegen sind
0, deswegen sind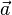 und
und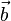 nicht orthogonal
nicht orthogonal
Vektorprodukt oder auch Kreuzprodukt
Mit dem Vektorprodukt rechnet man den Vektor aus, der zu 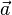 und
und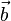 orthogonal ist
orthogonal ist

