Exponentialfunktionen.: Unterschied zwischen den Versionen
Enes12 (Diskussion | Beiträge) |
|||
| (29 dazwischenliegende Versionen von 3 Benutzern werden nicht angezeigt) | |||
| Zeile 3: | Zeile 3: | ||
Die Funktionsgleichung der Exponentialfunktion besteht aus folgender Form: | Die Funktionsgleichung der Exponentialfunktion besteht aus folgender Form: | ||
| − | f(x) = a<sup>x</sup> oder auch g(x) = c<math> \cdot</math>a<sup>x</sup> wobei: c <math> \in</math> <math>\R</math> | + | f(x) = a<sup>x</sup> oder auch g(x) = c<math> \cdot</math>a<sup>x</sup> wobei: c <math> \in</math> <math>\R</math>, a > 0, x <math>\in</math> <math>\R</math> ist. |
| + | Die Exponentialfunktion beschreibt für'' a > 1 einen Wachstumsprozess'' und für ''0 < a < 1 einen Zerfallsprozess''. | ||
| + | |||
| + | D.h.: a > 1 hat die Eigenschaft STRENG MONOTON STEIGERND und 0 < a < 1 hat die Eigenschaft STRENG MONOTON FALLEND. | ||
| + | |||
| + | <u>> Die natürliche Exponentialfunktion und ihre Ableitung:</u> | ||
| + | |||
| + | Die natürliche Exponentialfunktion f mit f(x)= e<sup>x</sup> hat die Ableitungsfunktion f ´mit f ´(x)= e<sup>x</sup>. Eine Stammfunktion ist F mit F(x)= e<sup>x</sup>. | ||
| + | |||
| + | ALLGEMEIN: | ||
| + | |||
| + | Ableitung: <br />f (x) = e<sup>v (x)</sup> | ||
| + | |||
| + | f ´(x)= e<sup>v (x)</sup><math>\cdot</math> v ´ (x) | ||
| + | |||
| + | Stammfunktion:<br /> F(x) = <math> \frac{1}{v'(x)} \cdot e^{v(x)} </math> | ||
| + | |||
| + | |||
| + | BEISPIELE: <br /> 1.) f (x) = e<sup>5x</sup><br /><br />f ´(x)= 5 e<sup>5x</sup><br /><br />F(x)= <math>\frac{1}{5}</math> e<sup>5x</sup>+c<br /> <br />2.) f(x)=5 e <sup>3x+7</sup><br /><br />f ´(x)= 5 e<sup>3x+7</sup><math>\cdot</math>3 = 15 e<sup>3x+7</sup><br /><br /> F(x) =<math>\frac{5}{3}</math> e<sup>3x+7</sup> | ||
| + | |||
| + | |||
<u>> Ableitung und Integrieren zusammengesetzter Funktionen:</u> | <u>> Ableitung und Integrieren zusammengesetzter Funktionen:</u> | ||
| + | |||
<u>> Untersuchung von Exponentialfunktionen:</u> | <u>> Untersuchung von Exponentialfunktionen:</u> | ||
| + | > Kurvendiskussion Anhand eines Beispieles:<br /> <br /> Funktion: f(x)= 5x<math>\cdot</math>e<sup>- 1/2x</sup><br /><br /> | ||
| + | 1.) Ableitungen:<br /> f ' (x)= 5<math>\cdot</math>e<sup>- 1/2x</sup> + 5x<math>\cdot</math>e<sup>- 1/2x</sup><math>\cdot</math>( - 1/2)<br />= e<sup>- 1/2x</sup> (5 - 5/2x)<br /><br /> f ' '(x)= - 1/2e<sup>- 1/2x</sup> (5 - 5/2x) + e<sup>- 1/2x</sup><math>\cdot</math>(- 5/2)<br /> = e<sup>- 1/2x</sup> (- 5/2 + 5/4x - 5/2)<br /> = e<sup>- 1/2x</sup> (5/4x - 5)<br /><br /> f ' ' '(x)= - 1/2e<sup>- 1/2x</sup> (5/4x - 5) + e<sup>- 1/2x</sup><math>\cdot</math>5/4<br />= e<sup>- 1/2x</sup> (- 5/8x + 5/2 + 5/4)<br />= e<sup>- 1/2x</sup> (15/4 - 5/8x)<br /><br />2.) Symmetrie:<br /> f ( -x) = 5<math>\cdot</math>(-x)<math>\cdot</math> e<sup>- 1/2<math>\cdot</math>(-x)</sup> = - 5x<math>\cdot</math>e<sup>1/2x</sup><math>\ne</math> f (x) --> KEINE ACHSENSYMMETRIE<br />f ( -x) = - 5x<math>\cdot</math> e<sup>1/2x</sup><math>\ne</math> - f(x) --> KEINE PUNKTSYMMETRIE<br /><br /> 3.) Nullstellen:<br /> notw. Bedingung f (x) = 0 <br /> 5x<math>\cdot</math>e<sup>- 1/2x</sup> = 0<br /> x = 0 <br /> N(0/0)<br /><br />4.) Verhalten gegen <math>\infty</math>:<br /><math> \lim_{x \to \infty}</math>5x<math>\cdot</math>e<sup>- 1/2x</sup> = 0 , weil 5x <math>\to</math> <math>\infty</math> und e<sup>- 1/2x</sup> <math>\to</math> 0<br /><br /> <math> \lim_{x \to -\infty}</math>5x<math>\cdot</math>e<sup>- 1/2x</sup> = <math> - \infty</math> , weil 5x <math> \to</math> <math>- \infty</math> und e<sup>- 1/2x</sup> <math> \to</math> <math> \infty</math><br /><br />5. Extremwerte: <br />notw. Bedingung f ' (x) = 0 <br /> e<sup>- 1/2x</sup><math>\cdot</math>(5- 5/2x) = 0<br /> Da e<sup>- 1/2x</sup> > 0 ist, braucht man nur die Klammer zu betrachten.<br /> 5 - 5/2x = 0<br />x = 2<br /><br /> hinreichende Bedingung f ' ' (2), um zu überprüfen, ob es Hoch bzw Tiefpunkte gibt: <br /> f' '(2) = e<sup>- 1/2<math>\cdot</math>2 </sup>(5/4<math>\cdot</math>2 - 5)<br />= e<sup>- 1</sup>( 5/2 - 5)<br />= - 0,92 < 0 daraus folgt: Es sind Hochpunkte vorhanden<br /> f (2) einsetzen, um zu überprüfen, wo der Hochpunkt ist<br />f (2) = 5<math>\cdot</math>2<math>\cdot</math>e<sup>- 1/2<math>\cdot</math>2</sup><br /><br /><math>\approx</math>3,68 d.h.: Hochpunkt (2 / 3,68)<br /><br />6. Wendestellen:<br /> notw. Bedingung f ' ' (x) = 0<br /> e<sup>- 1/2x</sup>( 5/4x -5) = 0 <br /> 5/4x -5 = 0<br />x = 4<br /><br /> hinreichende Bedingung: f ' ' '(4) um zu überprüfen, ob es Wendestellen gibt:<br /> e<sup>- 1/2<math>\cdot</math>4</sup>( 15/4 - 5/8<math>\cdot</math>4)<br /><math>\approx</math>0,17 <math>\ne</math> 0 d.h.: Wendestelle vorhanden<br /> bei: f (4)= 5<math>\cdot</math>4<math>\cdot</math>e<sup>- 1/2<math>\cdot</math>4</sup><br /><math>\approx</math>2,17 d.h.: Wendestelle (4/ 2,71)<br /><br />7. Graph: | ||
| + | |||
| + | [[Kategorie:Funktionen]] | ||
Aktuelle Version vom 17. Dezember 2010, 10:34 Uhr
> Eigenschaften der Funktion:
Die Funktionsgleichung der Exponentialfunktion besteht aus folgender Form:
f(x) = ax oder auch g(x) = cax wobei: c

, a > 0, x

ist.
Die Exponentialfunktion beschreibt für a > 1 einen Wachstumsprozess und für 0 < a < 1 einen Zerfallsprozess.
D.h.: a > 1 hat die Eigenschaft STRENG MONOTON STEIGERND und 0 < a < 1 hat die Eigenschaft STRENG MONOTON FALLEND.
> Die natürliche Exponentialfunktion und ihre Ableitung:
Die natürliche Exponentialfunktion f mit f(x)= ex hat die Ableitungsfunktion f ´mit f ´(x)= ex. Eine Stammfunktion ist F mit F(x)= ex.
ALLGEMEIN:
Ableitung:
f (x) = ev (x)
f ´(x)= ev (x) v ´ (x)
v ´ (x)
Stammfunktion:
F(x) = 
BEISPIELE:
1.) f (x) = e5x
f ´(x)= 5 e5x
F(x)=  e5x+c
e5x+c
2.) f(x)=5 e 3x+7
f ´(x)= 5 e3x+7 3 = 15 e3x+7
3 = 15 e3x+7
F(x) = e3x+7
e3x+7
> Ableitung und Integrieren zusammengesetzter Funktionen:
> Untersuchung von Exponentialfunktionen:
> Kurvendiskussion Anhand eines Beispieles:
Funktion: f(x)= 5x e- 1/2x
e- 1/2x
1.) Ableitungen:
f ' (x)= 5 e- 1/2x + 5x
e- 1/2x + 5x e- 1/2x
e- 1/2x ( - 1/2)
( - 1/2)
= e- 1/2x (5 - 5/2x)
f ' '(x)= - 1/2e- 1/2x (5 - 5/2x) + e- 1/2x (- 5/2)
(- 5/2)
= e- 1/2x (- 5/2 + 5/4x - 5/2)
= e- 1/2x (5/4x - 5)
f ' ' '(x)= - 1/2e- 1/2x (5/4x - 5) + e- 1/2x 5/4
5/4
= e- 1/2x (- 5/8x + 5/2 + 5/4)
= e- 1/2x (15/4 - 5/8x)
2.) Symmetrie:
f ( -x) = 5 (-x)
(-x) e- 1/2
e- 1/2 (-x) = - 5x
(-x) = - 5x e1/2x
e1/2x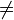 f (x) --> KEINE ACHSENSYMMETRIE
f (x) --> KEINE ACHSENSYMMETRIE
f ( -x) = - 5x e1/2x
e1/2x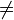 - f(x) --> KEINE PUNKTSYMMETRIE
- f(x) --> KEINE PUNKTSYMMETRIE
3.) Nullstellen:
notw. Bedingung f (x) = 0
5x e- 1/2x = 0
e- 1/2x = 0
x = 0
N(0/0)
4.) Verhalten gegen 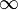 :
: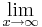 5x
5x e- 1/2x = 0 , weil 5x
e- 1/2x = 0 , weil 5x 
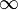 und e- 1/2x
und e- 1/2x  0
0
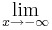 5x
5x e- 1/2x =
e- 1/2x = 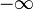 , weil 5x
, weil 5x 
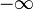 und e- 1/2x
und e- 1/2x 
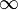
5. Extremwerte:
notw. Bedingung f ' (x) = 0
e- 1/2x (5- 5/2x) = 0
(5- 5/2x) = 0
Da e- 1/2x > 0 ist, braucht man nur die Klammer zu betrachten.
5 - 5/2x = 0
x = 2
hinreichende Bedingung f ' ' (2), um zu überprüfen, ob es Hoch bzw Tiefpunkte gibt:
f' '(2) = e- 1/2 2 (5/4
2 (5/4 2 - 5)
2 - 5)
= e- 1( 5/2 - 5)
= - 0,92 < 0 daraus folgt: Es sind Hochpunkte vorhanden
f (2) einsetzen, um zu überprüfen, wo der Hochpunkt ist
f (2) = 5 2
2 e- 1/2
e- 1/2 2
2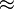 3,68 d.h.: Hochpunkt (2 / 3,68)
3,68 d.h.: Hochpunkt (2 / 3,68)
6. Wendestellen:
notw. Bedingung f ' ' (x) = 0
e- 1/2x( 5/4x -5) = 0
5/4x -5 = 0
x = 4
hinreichende Bedingung: f ' ' '(4) um zu überprüfen, ob es Wendestellen gibt:
e- 1/2 4( 15/4 - 5/8
4( 15/4 - 5/8 4)
4)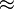 0,17
0,17 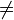 0 d.h.: Wendestelle vorhanden
0 d.h.: Wendestelle vorhanden
bei: f (4)= 5 4
4 e- 1/2
e- 1/2 4
4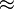 2,17 d.h.: Wendestelle (4/ 2,71)
2,17 d.h.: Wendestelle (4/ 2,71)
7. Graph:

