Prozeß- und Übergangsmatizen: Unterschied zwischen den Versionen
Aus KAS-Wiki
| Zeile 11: | Zeile 11: | ||
<math>A+B= \begin{pmatrix} a_1 & a_2 \\ a_3 & a_4 \end{pmatrix}+\begin{pmatrix} b_1 & b_2 \\ b_3 & b_4 \end{pmatrix}=\begin{pmatrix} a_1+b_1 & a_2+b_2 \\ a_3+b_3 & a_4+b_4 \end{pmatrix} </math> | <math>A+B= \begin{pmatrix} a_1 & a_2 \\ a_3 & a_4 \end{pmatrix}+\begin{pmatrix} b_1 & b_2 \\ b_3 & b_4 \end{pmatrix}=\begin{pmatrix} a_1+b_1 & a_2+b_2 \\ a_3+b_3 & a_4+b_4 \end{pmatrix} </math> | ||
| − | |||
=== Multiplikation: === | === Multiplikation: === | ||
| Zeile 18: | Zeile 17: | ||
<math>M*M=\overrightarrow\begin{pmatrix} a & b \\ c & d \end{pmatrix}*\downarrow\begin{pmatrix} e & f \\ g & h \end{pmatrix}=\begin{pmatrix} ae+bg & af+bh \\ ce+dg & cf+dh \end{pmatrix}</math> | <math>M*M=\overrightarrow\begin{pmatrix} a & b \\ c & d \end{pmatrix}*\downarrow\begin{pmatrix} e & f \\ g & h \end{pmatrix}=\begin{pmatrix} ae+bg & af+bh \\ ce+dg & cf+dh \end{pmatrix}</math> | ||
| + | |||
| + | <math>r*M=r*\begin{pmatrix} a & b \\ c & d \end{pmatrix}=\begin{pmatrix} ra & rb \\ rc & rd \end{pmatrix}</math> | ||
=== Inverse: === | === Inverse: === | ||
Version vom 1. Dezember 2009, 13:26 Uhr
Inhaltsverzeichnis |
Übergangsmatrizen:
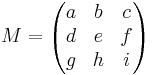

Eine Übergangsmatrix beschreibt einen Übergang von einer Dimension in eine andere.
Addition von Matrizen:
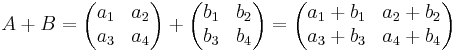
Multiplikation:
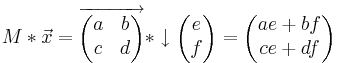
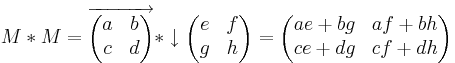
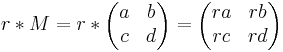
Inverse:
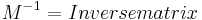
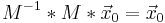
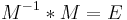
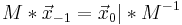


Grenzwert:
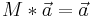
Ein Grenzwert ist dann erreicht, wenn sich die Matrix durch einen Vektor nicht mehr ändert z.B. bei Populationsentwicklungen. Wenn ein Grenzwert existiert, sind alle Spalten gleich, eine Gleichgewichtsverteilung besteht.
Einheitsmatrix:

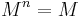
Nach n Jahren tritt wieder der gleiche Zustand ein, ist also zyklisch, wie bei Tierpopulationen.

